Death row cannot be a racial mirror of America, because blacks commit murder at a higher rate than whites - seven to eight times higher. Yet, the simplicity of Jackson's racial-mirror argument is appealing. Cleaned up to account for differential homicide rates, it becomes a powerful tool for assessing bias. This we have done and will now elaborate.

   Along the path to death row, race can insinuate itself at any point, from the prosecutor's decision to seek the death penalty, to the jury's verdict (recall O.J.) or recommendation of a death sentence. Of course, we could argue that sex or appearance might exert similar influences, and we would probably be correct.

   Chasing discrimination at every turn is burdensome and loaded with statistical pitfalls. Variables are many - controlling them a challenge. Even the choice of dependent variable may not be obvious. That is why we are indebted to Rev. Jackson. His racial-mirror argument considers none of this. It cuts to the bottom line: the color of death row. It disregards detail, seeing only the sum of bias on the road to execution. It is an "integral" approach, and asks only one question: Given black and white homicide rates, is death row too black, too white or just right? Uncorrected for differential murder rates, the answer is: too black. Corrected, the answers vary and may sometimes surprise.

The Color of Death Row without Bias
   Racial and ethnic groups behave differently in many ways. Crime is one of them, particularly violent crime, and specifically murder. Consider, for example, per capita murder-rates by race, derived from FBI and Census Bureau data and shown in Table 1.

   Suppose, in a given state, there are a total of N black and white inmates on death row. The probability, P_n , that n are black is given by the binomial distribution,

where p_B and p_W are the probabilities that a random inmate is black or white, respectively. We have isolated a black and white universe so that p_B + p_W = 1.

   Equation (1) is a yardstick for measuring racial equity. It describes death row as it would exist in a racially-neutral justice system. We can use it to find the probability of a particular racial mix on death row.

   More useful is the probability that the number of blacks (or whites) falls within a specific range. We obtain this by summing (1) over that range. Thus, the probability, P_ab , that the number of blacks on death row falls in the interval [a , b] is

   Another useful quantity is the most probable number of blacks on death row. This is the value of n that yields the largest P_n in (1). Because the binomial distribution is fairly symmetric, the most probable number of blacks (an integer) is very close to the average number of blacks (a non-integer). The average number of blacks is p_B N. For the cases we consider, we can without error take the most probable number of blacks to be p_B N rounded to the nearest integer.

   We cannot proceed much further without a value for p_B , the probability that a randomly selected inmate is black. This probability varies from state to state depending on the population mix. States with few blacks will have fewer blacks on death row. Rev. Jackson would argue that p_B is the black population fraction of the state, but of course that is not so. The probability, p_B , is the black fraction of capital murderers, a vastly different number.

   For convenience, assume that the black fraction of capital murderers is equal to that of other murderers. Let N_BM be the number of black murderers and N_WM the number of white murderers in a given state. Then for that state,

   We define the per capita black to white murder-rate ratio, R ,

where N_B and N_W are the black and white populations, respectively, in the state. Evidence suggests that criminal behavior and consequently R , is more characteristic of race than of geography. In fact, data show R to be essentially invariant to crossing state boundaries, its value falling between 7 and 8. That is, a black is between 7 and 8 times more likely than a white to be a murderer. The quantity, R, may be evaluated from population and crime databases.

   Because the per capita black to white murder-rate ratio, R , is nearly constant, we find it convenient to express p_B in terms of it. From (3) and (4) we write

   Though R remains constant, the population ratio, N_W / N_B , varies by state. In Figure 1, using a value of 7.5 for R, we see how the probability of a random death-row inmate being black varies with a state's population mix.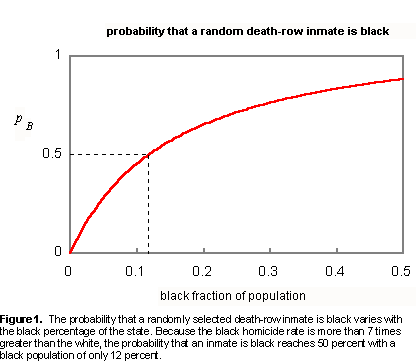

Is Death Row Too Black?
   We illustrate how to test for bias on death-row using data from Alabama. We consider only non-Hispanic whites and non-Hispanic blacks. Accordingly, the terms white and black, as we use them below, do not include Hispanics of any race.

   As of April 1, 2000, Alabama held under sentence of death 97 whites and 86 blacks. Following Rev. Jackson, we observe that African Americans are 26 percent of Alabama's population, but 47 percent of death row. (Our numbers are more recent and thus differ a bit from Jackson's. The newer data are even more objectionable from his vantage.) But is Alabama's death row really too black as Jackson alleges?

   Using in (5) a value of 7.5 for the black to white per capita homicide ratio, R, we find the probability, p_B , of a random death-row inmate in Alabama being black to be 0.729. That is, "on average" we should find 72.9 percent of Alabama's death row to be black. If the Alabama justice system were bias free, the most probable number of blacks in its 183 prisoner death-row would be (0.729)(183) or 133. With only 86 blacks under sentence of death, Alabama's death row is too white -- much too white!

   How unlikely is Alabama's death row? Statistical fluctuations normally produce clustering about most probable values. In statistical terms, the best question to ask is how many standard deviations (SD) from the most probable number of blacks (133) is the actual number (86)? The standard deviation, σ, of a binomial distribution is given by

For Alabama, N = 183 and p_B = 0.729, yielding σ = 6.01. Thus the number of blacks on death row is (133-86)/6.01 or 7.8 SD away from the most probable number (133). The likelihood of finding this number of blacks (or fewer) on death row by chance is 1 in ~10¹⁴. Not only do we find the sentence of death in Alabama to be biased against white suspects, it is extraordinarily so.

   We subjected each state, with a death penalty, to similar analysis. In some states a small black population precluded a meaningful statistical analysis. (For example, only 1 in ~1700 Idahoans is black.) Still other states have death rows too small or with too few blacks to obtain useful results. For those states meeting the statistical requirements, we summarize our findings in Tables 2, 3 and 4.

   How then did we find a pattern of discrimination against white suspects? Do our results conflict with those of Baldus et al? Not at all. They are perfectly compatible. Because whites mostly kill whites and blacks mostly kill blacks, if white victims are valued more, white perpetrators will be more likely to get a death sentence and end up on death row. We might say that white suspects pay the price for discriminating against black victims. The eminent sociologist, Steven Goldberg, observes that eliminating discrimination against black victims would have the paradoxical effect of increasing the percentage of black murderers sentenced to death.

Lawyers and Mathematicians: Two Perspectives
   The Legal Defense Fund of the NAACP says of the Baldus study,

"After reviewing over 2500 homicide cases in Georgia in the 1970's, and having controlled for 230 non-racial factors, the study concluded that a person accused of killing a white was 4.3 times more likely to be sentenced to death than a person accused of killing a black."

   And from the ACLU:

"Professor David Baldus examined sentencing patterns in Georgia in the 1970's. After reviewing over 2500 homicide cases in that state, controlling for 230 non-racial factors, he concluded that a person accused of killing a white was 4.3 times more likely to be sentenced to death than a person accused of killing a black."

   The NAACP and ACLU are working from the same script. It is a script used by many death-penalty opponents, because they all take their cues from the same source: the Supreme Court ruling in McCleskey v. Kemp (1987). Justice Powell wrote for the majority:

"One of [Baldus'] models concludes that, even after taking account of 39 nonracial variables, defendants charged with killing white victims were 4.3 times as likely to receive a death sentence as defendants charged with killing blacks."

   But the Court made a boo boo. After so many years, death-penalty opponents should know that the Supreme Court confused odds with probability. (For more about this, see Arnold Barnett's delightful article, How Numbers Deceive.) Baldus found that the odds (not the probability) of receiving a death sentence were 4.3 times greater for a defendant charged with killing a white than for killing a black.

   Odds are defined as probability divided by (1 minus probability). If you do the arithmetic, you will find that the probability of a death sentence for killing a white victim, P_WV , is not 4.3 times the probability, P_BV , for killing a black. The two probabilities are related as follows:

   Figure 3 graphically illustrates 1) what Baldus meant, 2) what the Court said he meant, and 3) how each relates to bias-free justice.

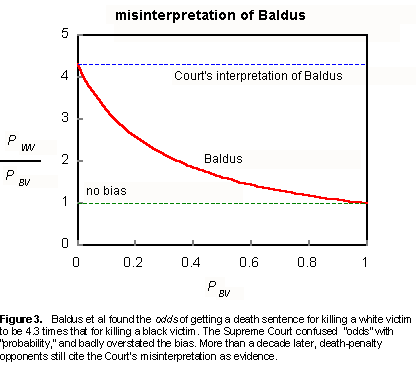   From Figure 3 we see that the probability of receiving a death sentence for killing a white victim was greatly exaggerated by the Court. Only when the chance of a death sentence is very small (i.e., not many aggravating circumstances) is the factor of 4.3 approached. But since the death penalty is not likely to be imposed under these circumstances, the victim's race becomes moot. None of this is to say that the victim's race is unimportant. In the gray area where the death penalty is neither very likely nor very unlikely, it is a factor, not as big as the Court supposed, but enough to account for the bias observed in the South. There is no need for the NAACP, ACLU and others to build on the Court's error.

   More than a decade has elapsed since McCleskey v. Kemp, yet the NAACP and ACLU continue to trumpet the Court's false conclusion. They entertain not only false, but dishonest uses of the sources from which they draw support. We harbor no such inclinations, seeking only to clarify, and to communicate the results of our inquiries to a world thirsty for Griffian knowledge. Being thus limited, questions remain unanswered -- two in particular: Why is the South unique among regions for death-row discrimination? And, what is it about Pennsylvania, that it stands alone among states by discriminating against black homicide suspects? We invite the reader to contribute $0.02.